Introduction

It is characteristic of society today that any area of thought, belief or action is viewed in a dualistic manner, either positively or negatively. Much of the dynamic of society is determined by interaction, competition or conflict between those holding such polarised perspectives on any issue. The structures currently available reflect this situation by either:

1. focusing attention on one pole of the duality, ignoring the other, or treating it with varying degrees of hostility, or
2. integrating the duality into the structure and permitting a change over time in response to a majority (such as at elections) between acting in terms of one pole or of the other, or
3. integrating the duality into the structure such that one division favours the approach indicated by one pole of the duality and another favours that of the second pole. Since the dualistic approach is extremely divisive, the question is whether more adequate approaches are not available as a basis for new kinds of structures that could by-pass the conflict situation built into the dualistic approach without denying its reality.

The purpose of Part 1 of this article is to draw attention to the manner in which this problem has been examined in architecture and how dualities are balanced and transcended within what are known as "tensegrity" structures (1). Such an approach suggests a number of interesting possibilities by which to handle:

1. sets of dualities, such as opposing values or viewpoints,
2. groups pursuing opposing objectives,
3. relationships between structures based on the "organic, wholistic" view that "all men are brothers" and the "bureaucratic" view which emphasizes the importance of "rational, effective" structures, and
4. relationships between an organizational system and any corresponding (or complementary) network.

The question is whether this approach offers us clues to a new kind of "psycho-social architecture". This is explored in Part 2 of the article.

The Lesson from Building Design

It would be desirable to precede this section by a development of the familiar argument that there is an interplay between the principles governing the physical architecture and designed structures current in a society and those governing the psycho-social organizations of that society. Unfortunately space limitations preclude doing so in any adequate manner and it is only possible to develop the following argument in such a way as to stress that correspondence.

A building, like any other structure (including psycho-social structures) has forces acting on it, or stresses acting within it, which are trying to deform it or cause it to move. These forces pass through the structure, pushing on some elements (hence "compressive" force) and pulling on others (hence "tensile" force). Until the last century, most of the building materials available were effective in resisting compressive forces (e.g. brick, stone), but few durable materials were capable of withstanding even moderate tensile stress (with the limited exception of wood). Consequently buildings and bridges were designed so that large tensile stresses did not occur in them.

The fundamental problem in building is to support a load, whether in the case of a bridge or simply to establish a covered space. As in Diagram 1 this can be done with beams on columns. But in order to increase the floor space and reduce the materials required for a given load, arches of increasing sophistication have been constructed from Roman times.

"The arch is essentially a device for dispensing with a center post, by sp/itting the thrusts a center post would support and by deflecting them to the sides. The downward pull of gravity on the keystone is converted into paired outward thrusts, which the face angles of successive stages transform into downward thrusts once more, but downward thrusts now borne by the side columns. Thus the columns actually support the weight of the keystone and its neighbours, without having to be located directly under the stones whose weight they bear. So a central space is cleared beneath the arch.

It is clear that everything is held in place by weight, so that the continuities of stress are chiefly compressive. Two or more intersecting arches will define a dome-shaped space, again clear of supporters because the work of support has been transferred to peripheral columns...

Though the visible continuities are compressive, there is in fact an invisible tension network which analysis cannot ignore. Each component of a stone dome is held in place by the earth's gravitational field, pulling tensionally "downward" through the structure. If the dome were inverted, the force that pulls it together would pull it apart. If it could be placed in orbit, it would drift apart. Thus its structural integrity depends on the weight of its components, and on the way they are oriented in earth's gravitational field. A successful design is essentially a feat of balancing. All forces are resolved along lines perpendicular to earth's surface, so that gravity and the mutual impenetrability of stones achieve a standoff. Any forces that deviate from this system of perpendicular resolutions will create a tendency to collapse inward or outward, and must be counteracted by braces or buttresses". (Kenner, pp. 3-4).

As indicated above, the diagram shows how such buttresses may work (2). In the absence of buttresses, some form of steel band may be wrapped around the structure to keep it together at its region of potential failure (a chain is used around the dome of St Peter's in Rome). The necessity for such devices, designed with great precision by the engineers of today, makes clear how precarious is the structure's equilibrium, even when equilibrium is achieved without their aid.

This approach continues the tradition established in Roman times. Even in the past century, with the development of new high tensile strength materials (e.g. alloys), few structures have been designed to exploit them. In the sophisticated structures of nature, such as trees for example, there is however always a balance between the use of compression and the use of tension- and the compressive elements are usually much bulkier (to prevent buckling) than the tensile elements.

As a consequence of this observation a completely different approach was suggested by R. Buckminster Fuller and here described by Hugh Kenner:

"The way to do this is to abandon altogether the concept of structural weight impinging on the compressive continuity of bearing members, the whole guarded by occasional tensional reinforcement. Instead of thinking of weight and support, we may conceive the domical space enclosure as a system of equilibriated omnidirectional stresses. Such a structure will not be supported. It will be pulled outward into sphericity by inherent tensional forces which its geometry also serves to restrain. Gravitation will be largely irrelevant...

Unlike the stone arch or the stone dome, such structures are not made stronger by being made heavier. In fact, they can with advantage be made negligibly light in comparison with the tensional forces that bind the components. The one-way tension of terrestrial gravity is replaced by the multi-direction tension of structural members. The system is therefore stable in any position.

Moreover, a tendency to peripheral or local stresses, such as those restrained by the chain around the dome of St Peter's, is supplanted by a multi-directional stress equilibrium. A corresponding multidirectional tension network encloses accidental stresses wherever they arise. There are no points of local weakness inherent in the system". (Kenner, pp. 5-7).

In such a system, the tensional integrity ("tensegrity") for the transmission of forces throughout the structure is achieved through a continuous network of tensile elements, the compression elements being discontinuous in a soap bubble or a balloon, an envelope of surface tension attempts to close inward against the outward compressive force of the enclosed air. The equilibrium modelled as a spherical shape whose symmetry is complete. In a model of a tensegrity system (see Diagram 2), the struts push outward like the air inside a balloon, and the tendons pull inwards like the skin of a balloon. Increasing the forces in either the balloon or the tensegrity system increases the strength and load-bearing capacity of the system (3).

Systems and Polyhedra

There are two ways in which an operational space can be elaborated:

1. The space is first conceived as distinct from its external environment. Fuller states: "A system is the first subdivision of Universe... Oneness, twoness, and threeness cannot constitute a system" (p. 95-96). Also: "Systems are unpredicted by oneness, twoness, or threeness" (p. 98). Namely it takes a minimum of four vertexes to define a set of planes giving insideness and outsideness, the basic requirement to ensure that a system in distinguished from its environment (4).

In this approach, one commences with a whole (perhaps conceived as a spherical surface), and having broken it up by establishing a minimum of 4 vertexes, an independent system is achieved. Further vertexes can now be added to complexity the system, if this is appropriate to whatever it is supposed to represent (5).

2. Structural elements (struts) can be taken and it is found that an enclosed space is not defined until 6 such elements meet at 4 vertexes. The shape of the space can be rendered more complex using more elements. Again, a system is defined.

The seemingly simplistic distinction between these two approaches has been made because the first involves essentially the progressive conceptual breakdown of a whole, whilst the second proceeds in terms of the aggregation of parts.

With regard to the systems which emerge by either procedure, Fuller makes the unexpected point that: "A/l systems are po/yhedra" (p. 95). He argues that the focal points for energy events in any system are linked into a closed pattern of relationships that can effectively be represented by an appropriate polyhedron (6). "Polybedra are topologically describable finite system enclosures", (p. 655).

Symmetry and Sphericity

Since systems can be represented by polyhedra, two questions raised by both approaches are:

1. how symmetrical is the space defined and
2. how closely does it approximate to a sphere.

The first is important because: "Symmetry is a measure of a system's ability to absorb rearrangment that cannot be detected " (Kenner, p. 54).

"Ability to respond as a system means that local stresses are being uniformly transmitted throughout the structure, and uniformly absorbed by every part of it. The system's symmetry is not deformed: the system expands as a whole or contracts as a whole. This is not the behavior we are used to in any structures of our previous experiences. The compression members do not behave like conventional engineering beams... Ordinary beams deflect locally. The tensegrity "beam" does not act independently of "the whole building" which contracts only symmetrically when the beam is loaded. The tensegrity system is synergetic - a behavior of the whole unpredicted by the behavior of the parts" (Fuller, p. 401).

"This principle points toward valuable economics in material. Instead of designing every part of the system to receive unassisted whatever loads it may incur, with consequent local accretions of weight and bulk, we may instead design the system on the assumption that local stress will be transmitted throughout its extent and shared by all its members. The normal state of the system is not a state of rigidity but a state of equilibrium, to which, when disturbed, it seeks to restore itself" (Kenner, p. 12). The greater the symmetry, the greater the ability to distribute and absorb local stresses throughout the whole system.

The question of the sphericity of the system is important as an indication of the efficiency with which energy may be distributed throughout the system. This is maximised when the planes of symmetry of a polyhedron constitute great-circles on a circumscribed sphere. Tensegrity systems, which are supported by tension are most economical when the tensile network runs for considerable distance without changing direction, namely when the vertices are aligned along great-circle axes. In Fuller's words:

"Compound curvature, or sphericity, gives the greatest strength with the least material... Not until we have three noncommonly polarised, great-circle bands providing omnitriangulation as in a spherical octahedron, do we have great circles acting structurally to self-interstabilize their respective spherical positionings by finitely intertriangulating fixed points less than 180 degrees apart... The more minutely the sphere is subtriangulated by great circles, the lesser the local structural-energy requirements and the greater the effectiveness of the mutual-interpositionina integrity. This spontaneous structural self-stabilizing always and only employs the chords of the shortest great-circle arc distances and their respective spherical finiteness tensional integrity... With each increase of frequency of triangular module subdivisions of the sphere's unitary surface, there is corresponding increase in the fail-safe advantage of the system's integrity" (Fuller, p. 383).

Symmetry and sphericity therefore blend together in the quest for spherical symmetrical systems, when energy transmission efficiency and stress absorption capacity are the issue. Of all the polyhedra, however, only three (and their derivatives) provide prime symmetry systems: tetrahedron, octahedron (and cuboctahedron) and icosahedron (and icosidodecahedron). The icosahedron, with 31 great circles, has the highest number of identical and symmetric exterior triangular faces of all the symmetric polyhedra defined by great circles (tetrahedron has 4, octahedron: 13).

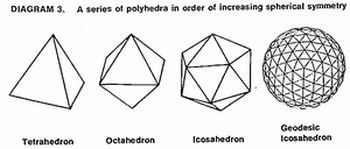
Returning to the elaboration of a system, it is the tetrahedron (4 vertices, 6 edges) which constitutes the minimum single symmetrical system, containing the least volume with the most surface (7). The sphere, in contrast, encloses the most volume with the least surface.

A system of this type, because of its relatively poor sphericity, could be said to be less efficient than the icosahedron type. The challenge in developing (or "maturing") a system might then be to make it as closely approximate to a sphere as possible, with an appropriate polyhedron. However, because the representation of systems by polyhedra has hardly been explored, except by Fuller, it is not clear which polyhedra-based systems would be useful under what circumstances-particularly in the case of psycho-social systems. (N.B. Basic polyhedra, and their relationship to tensegrity systems, are described on pp. 254-255).

Significance of Tension and Compression

In looking for alternative structures, it is obviously not a question of proceeding with an attitude of "compression, bad; tension, good", Fuller himself stresses the intimate relationship between the two: "No tension member is innocent of compression, and no compression member is innocent of tension... Tension and compression are inseparable and coordinate functions of structural systems, but one may be at its most prominent phase, while the other is at its least prominent phase" (p. 357, see also footnote 8)

He does however make the distinction that: "So we find the compression complexes tend to do the small local structure/ tasks in Universe, and the tension complexes tend to do the large structure/ tasks in Universe. As tension accounts for the large patternings and pattern integrities, compression trends into locally small pattern integrities" (p. 357 and footnote 9).

The distinction between "compression" and "tension" and their full meaning elude precise definition because of their fundamental nature as analyzed by Fuller. Every attempt at definition succeeds only in presenting some characteristics but not all. And to the extent that a definition succeeds in encompassing a greater number of characteristics, the special nature of the abstraction required tends to render the statement incomprehensible. (The problem is similar to, if not identical with, the Yin-Yang concept in Chinese philosophy that is concerned with fundamental twoness; definition is itself a yang-type process.)

The difficulty is compounded because an explanation can only be adequately given in terms of a specific system within which the relationship between a minimum of six such elements must be discussed. The concrete models of the tensegrity systems of interest here are constructed with "struts" and "tendons". But the compressive struts also represent vectors (10), and the tendons represent tensors. For Fuller: "Vectors and tensors constitute all e/ementary dimension. A vector represents an expelling force (from the system) and a tensor an impelling force", (Fuller. p. 260).

The polyhedron by which the system is represented is delineated with lines (11) crossing at points (12). For Fuller: "Lines are vector trajectories" (p. 260) and "Every trajectory in a system will have to have at least two crossings", (p. 271).

The reader should refer to Annex 3 which illustrates the nature of tensegrity structures. If additional compressive elements (struts) are added appropriately, the degree of approximation to a sphere increases progressively. The tensile elements outline the sphere's outer surface (to the extent that the polyhedron approximates to it), whilst the struts outline the inner surface. The integrity of the spherical skin as a whole is independent of central support. It does however require that all tensional circuits be completed (possibly by anchoring a truncated structure to the earth, as in most geodesic domes).

The first principle of geodesics as stated by Hugh Kenner is: "The structure is lifted outward by a hidden tensional system. It resembles a contained explosion, like a balloon: compressive out-thrusts held in a tensile web, with this difference that the forces pulling outward are also coming from the web. The structural members are not falling in together, nor in any important way leaning on one another" (p. 43).

Fuller emphasises that the behaviour of the individual compressive elements cannot be understood in isolation or in terms of a simple chordal two-way thrust: "The comprehensive spherical tensor network can only relax inwardly. When all in place, the tensegrity compression struts can only prevent the tension network from closing inward toward the sphere's center, which is its comprehensive proclivity. The synergetic force of the struts (that is, their total interrelationship tendency) is not predicted by any one strut taken singly. It is entirely omniradially outward. The force of the strut is not a chordal two-way thrust" (p. 386). The compressive element "is not fastened in shove or sheer. It pulls outwardly of the spherical system, away from the tension members at both of its ends simultaneously; When released, it pops only outwardly from the sphere's center" (p. 390). It does not shove by the tension member from which it is released.

Preliminary conclusion

In the light of the above generalised description of tension and compression in tensegrity systems, the challenge is to see whether the intriguing clues can be used to clarify the nature of any parallel in psycho-social systems, especially organizations (see Part 2, pages 258-265).

In so doing it should be remembered that tensegrity systems exist and can be comprehended as wholes, even though they may be complex (Diagram 2). Extensive verbal descriptions may in fact only serve to disguise the wholistic, synergistic qualities of such systems -- hence the value of constructing and handling models, which may then serve as a scaffolding for reflections about their psycho-social equivalents. (The above paragraphs should be re-read in the light of any resulting insights)

Annex 2: Summary of derivation of tensegrity structures (tentative)

Based on  (q)	Platonic polyhedra  (k)	Archimedean polyhedra  (j)	<	Geodesic	polyhedra  (m)		Other polyhedra  (c)	Various hybrids  (d)
.	.	.	Alternate	subdiv.	Triaconic	subdiv.	.	.
.	.	.	Platonic	Archimed.	Platonic	Archimed.	.	.
Diamond pattern (h)	.	.	(e)	(e)	.	.	.	(g)
Circuit pattern (a,f)	(j)	(j)	(n)	(n)	.	.	.	(g,h)
Zigzag pattern	.	(p)	.	.	.	.	.	.
Other spherically symmetrical patterns	.	.	.	.	.	.	.	.
Non-spherically symmetrical patterns (b)	.	.	.	.	.	.	.	.

 (a) Enantiomorphic (left and righthand version)

(b) Examples: 2-D (flat), simple non-spherical structures multilayer), ellipsoid, joined (by matching surfaces masts or trusses, as grids or skeletal structures)

(c) Other polyhedra may be grouped as fellows, although few have (as yet) lent themselves to use for tensegrily systems: other facially regular convex polyhedra (92), Archimedian duals (13), prisms/anti-prisms (and duals), Kepler-Poinsot and related polyhedra, irregular Dolvhedra.

(d) Obtained by adding extra elements, eliminating or combining elements, or adjusting the relationship between elements. (One structure can be converted into another in this way). Includes simple structures (spherically symmetrical) with elements through the centre.

(e) Diamond patterns tend towards circuit patterns with the increase in the number of elements characteristic of geodesics. Tensile force maybe carried by the outer surface of the elements in compression thus eliminating the distinction between dimaond and circuit.

(f) Compressive circuits may each be made up of:

1. 3 (triangles), 4 (squares), or more elements separate but Interwoven,
2. the centres of such planar circuits may be identical with that of the sphere, or towards the circumference,
3. alternatively, there may only be one continuous circuit, linking all compressive elements, which weaves about without touching itself
4. a given figure may contain circuits with differing numbers of elements.

(g) Diamond-pattern systems can be derived from circuit-pattern systems and vice versa.

(h) Diamond-pattern based systems can be described in terms of the number of elements and layers of which they are composed. Most of the larger structures are cylindrical and not spherical.

(j) Circuit-patterns can only be based on uniform polyhedra bounded by great circles and with four edges Per vertex. These are: octahedron, cuboctahedron and icosidodecahedron The three prime symmetry systems from which these are derived are: octahedral, icosahedral and tetrahedral lusuallv inefficient". The octahedron itself does not give rise to a cricuit-pattern tensegrity, only its geodesic subdivisions. Lesser circle systems (small rhombicuboctahedron and small rhombicosidodecahedron) have no lines of truncation for domes.

(k) Platonic polyhedra are: tetrahedron, hexahedron (cube), octahedron,

(l) Archimedean polyhedra are: cuboctahedron, truncated tetrahedron, truncated octahedron, truncated cube, small rhombicuboctahedron, great rhombicuboctahedron, snub cube, truncated icosahedron, truncated dodecahedron, icosidodecahedron, great rombicosidodecahedron, snub dodecahedron, small rhombicosidodecahedron

(m) Based on subdivision of faces of the principle polyhedra to produce a more spherical figure. Only the two most common methods of subdivision are cited: alternate and triacon. Such methods may also be applied to polyhedra other than Platonic and Archimedean. To reduce complication the tendency is to use Platonic polyhedra, only, with faces subdivided as many times as appropriate. All but the 2 snub Archimedean figures are in fact generated by 2 to 12 frequency divisions of the Platonic polyhedra

(n) Circuit-pattern systems can only be based on polyhedra whose triangulated faces have been subdivided to a frequency which is a multiple of two. Zigzag patterns are only possible for frequencies which are a multiple of three. In both cases several versions of any given figure result according to whether elements of equal or of different length are used (the latter gives the least distorted result). Most geodesics are based on frequency subdivisions of the octahedron or the icosahedron.

(p) Of the 13 Archimedean figures, 7 have 3 edges meeting at a vertex and form the basis for a tensegrity system.

(q) The valency of a tensegrity is the number of tensile elements attached to the end of each compression element. A minimum of 3 is required for a 3-D figure (and gives rise to tensegrity prisms, for example). The simple valence-4 spherical tensegrity is based on the octahedron; the two others are the cuboctahedron and icosidodecahedron. Valence-5 occurs with the icosahedron. Valence-6 is characteristic of geodesic subdivision.

Annex 3: Geodesic polyhedra generation

The basic polyhedra have too few edges to construct a spheric tensegrity system of large diameter without the structural elements having to be excessively long. Geodesic polyhedra, having the spheric surface broken up into as many smaller size faces as are required (thus requiring shorter structural elements), may be produced as follows, by the "alternate method" -- after any non-triangular faces on the basic polyhedron have been divided into triangles, before the frequency sub-division is applied. The resulting triangular faces of the geodesic polyhedra, such as those shown below, can then be grouped together in patterns at triangles (1), diamonds (2), squares (4), pentagons (5), or hexagons (6), depending on the subdivision frequency and the original polyhedron used. If the subdivision frequency of the original polyhedral triangular face is a multiple of two, these patterns can then be used for tensegrities based on the "circuit pattern"; if a multiple of three, on the "zigzag pattern"

Annex 4: The Spheric Experience

Thirty spokes share the wheel's hub;  It is the centre hole that makes it useful.  Shape clay into a vessel;  It is the space within that makes it useful.  Cut doors and windows for a room;  It is the holes which make it useful.  Therefore profit comes from what is there;  Usefulness from what is not there.

 

"What we do have experimentally as a sphere is an aggregate of energy event foci approximately equidistant in approximately all directions from one energy-event focus. This is a system in which the most economical relationships between embracingly adjacent foci are the great-circle chords, and not the arcs". (Fuller, p. 654)
"The spheric experience is a high-frequency, omnidirectional complex of events and their relatedness. Since it is concerned with the most economical relatedness, we can also speak of it as a geodesic spherical experience. This is where the importance of chords comes in. A chord is abstract, yet tensive. A chord has pull: we would probably not think about the connections unless there was some pull between them. The function of the chords is to relate. The event is the vertex. The reaction is the chord, the pulling away. And the resultant is the inadvertent definition of the nothingness of the area and volumetric spaces... Areas and volumes are incidental resultants to fionding the connections between events of experience" (p. 656)
"Almost-spherical polyhedra are the nearest approximation (to a sphere). It can only be treated with as polyhedral - as an aggregate of points in which the most economical relationships are chords; ergo, geodesics". (p. 654)

"..since physics has found no continuums, we have had to clear up what we mean by a sphere. It is not a surface; it is an aggregate of events in close proximity. It isn't just full of holes; it doesn't have any continuum in which to have holes. The word polyhedron has to go because it says "many-sided", which implies a continuum. We don't even have the faces. Faces become spaces. They become intervals. They become nothing. The Einsteinian finite Universe - an aggregate nonsimultaneous Universe - is predicated only on the absolute finiteness of each local energy-event package and the logic that an aggregate of finites is itself finite". (p. 655)

"Zero and the concept of emptiness, too, are comparatively late inventions (clearly because they too leave nothing to hold onto in explaining them). Even now we find it hard to conceive of emptiness as such: we only manage to think of it as the absence of something positive. Yet in many metaphysical systems, notably those of the East, emptiness and absence are regarded as more fundamental and ultimately more substantial than presence. This is also connected with the fact, now acknowledged by most biologists, that symmetry, being the natural condition of an unstressed situation, does not require explanation, but on the contrary it is asymmetry which needs to be explained." (Christopher Alexander. Notes on the Synth-his of Form. Harvard University Press, 1971, p. 197)

Notes and References

1. There are few sources of information on tensegrity, originally investigated by R. Buckminster Fuller. This article reviews the insights in the three principle sources currently available, although their authors are not responsible for any interpretations here of the possible psychosocial implications of these principles: R. Buckminster Fuller. Synergetics: explorations in the geometry of thinking. Macmillan, 1975 (Tensegrity, pp. 372-432). Anthony Pugh. An Introduction to Tensegrity. University of California Press, 1976 (See also: Polyhedra; a visual approach Same author and publisher). Hugh Kenner. Geodesic Math and How to Use It. Los Angeles, Uni varsity of California Press, 1976 (Part 1: Tensegrity, pp. 3-44).

2. The above quotation should be re-read in the light of the psychosocial implications argued in the following sections. There is an intriguing parallel between the keystone and the position and function of the leader (or "boss") in an organization.

3. It has calculated that a geodesic metal sphere approx. 0.5 miles in diameter would drift like a bubble if the air inside it were one degree warmer than that outside.

4. "Not only can there be no awareness until there is otherness to be aware of, but there can be no magnitude awareness with only one otherness... You can have no sense of awareness of shape with just one otherness or two othernesses. Shape awareness commences only with three othernesses where the relationship of three as a triangle has finite closure. Shape is what you see areally and until there is closure, there is no area of otherness. Not until we have four otherneses do we have macrocosmic volumetric awareness. Four is required for substantive awareness. System awareness begins when we find the otherness surrounding us, when we are omnidirectionally enclosed." (Fuller, p. 656)

5. With regard to this approach, a special algebraic / logical notation has been developed: G. Spencer Brown. Laws of Form. Allen and Unwin, 1969.

6. "All the interrelationships of system foci are conceptually represented by vectors. A system is a closed configuration of vectors. It is a pattern of forces constituting a geometrical integrity that returns upon itself in a plurality of directions" (Fuller, p. 97).

7. "By tetrahedron, we mean the minimum thinkable set that would subdivide Universe and have interconnectedness where it comes back upon itself. The four points have six interrelatednesses" (Fuller, p 333)

8. "Functions occur only as inherently cooperative and accommodatively varying aspects at synergetically transforming wholes... The meaning of function is that it is part of a complementary pattern... Tension and compression are always and only interfunctioning covariables whose seeming relative importance is a consequence of local pattern inspection" (p 359).

9. "The sum of all the interactive-force relationships of Universe must continually accelerate their intertransforming in such a manner as to result in ever more remotely and locally multiplied islanded compressional functions - comprehensively cohered by ever-enlarging finite patternings of the tensional function" (p. 360). In our societies, large agencies (compressional complexes ?) tend to be linked with various kinds of liaison networks (tensional complexes ?) which cross jurisdictional boundaries, thus ensuring a minimum of coordination.

10. "A vector manifests a unique energy event-either potential or realized -expressed discretely in terms of directions, mass, velocity, and distance... A vector always has unique direction relative to other events. It is discrete because it has a beginning and an end. Its length represents energy magnitude, the product of its velocity and its mass. The direction is angular in respect to the axis of reference of the observer or in respect to an omnidirectional coordinate system." (Fuller, p. 259).

11. "Speaking operationally, lines are the products of the energy interactions of two or more separate systems. The local environment is system. A line is always formed by an alteration of the local en vironment by another system. "Lines" are the patterns of consequences of one system altering another system either by adding to it or taking away from it." (p. 252).

12. "What we really mean by a point is an unresolved definition of an activity... Without insideness, there is no outsideness; and without either insideness or outsideness, there is only a locus fix... A point's definitively unresolved event relationships inherently embrace potential definitions of a complex of local events. When concentrically and convergently resolved, the point "proves" to be the "center" -- the zero moment of transition from going inwardly and going outwardly. Physical points are energy-event aggregations." (p. 257-8).