Introduction

When a decision is made to pursue a group of concerns, there is a basic problem of ensuring that they are appropriately interrelated and do not simply constitute a fragmented collection of initiatives. This paper examines a new approach to the systematic recognition of the interrelationships necessary to the emergence of a viable configuration of concerns at a new level of significance.

The "concerns" could take many forms. They could emerge from individual resolutions or recommendations. They could be elements of a declaration of principles. Or they could be research priorities, projects, or the functions required in an organization. That they are interrelated, or at least should be, follows from the initial intention whereby they were treated as a set of in some way complementary elements (1).

How they are interrelated is seldom clarified initially and often only emerges, if at all, in the operational considerations in any organization(s) through which they are implemented. The number of concerns accepted in a given case depends both on the distinguishing power and on the ability to comprehend and communicate their necessary nature as a consequence of that act of distinction. The level of ability may not be adequate for a viable structure to emerge (1).

Structural options

Leaving aside situations in which no conscious attempt is made to structure such relationships, one of two structural extremes may be used to reflect them:

• hierarchy (or tree) structure, as in conventional organisations e.g. for 6 functions (see Fig. 1).
  
  
  
• associative network structure, as in "network"organisations e.g. for 6 functions (see Fig 2)
  
  

The first of these merely indicates formal reporting channels and not the necessary operational communications. It is also in many ways symptomatic of the problems to be overcome. The second is seldom used explicitly, even though operational links could be represented, but it also fails to clarify what are necessary relationships. A more recent innovation also fails in this respect:

• matrix structure, as in "matrix" organizations (see Fig 3)

Fig. 3	Dept A	Dept B	Dept C	Dept D
Administration	X		X
Coordination	X	X
Prog. develop.			X	X

In previous papers the advantages of investigating "tensegrity" organisation have been put forward (2, 3). The problem of deciding on functions and relating them to a given tensegrity pattern was raised but not addressed. This is the purpose of the next section.

Recognizing relationships

The question is how to represent explicitly the relationships perceived in a set of functions which are to be the basis of a viable organisation, policy, programme or conceptual scheme(1). One interesting possibility is to adapt the conventions of the Venn diagram used in symbolic logic and "new mathematics".

e.g. for 3 functions (see Fig. 4)

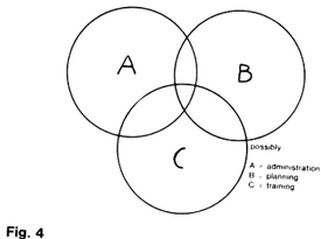It is the fact that functions A and B are represented as intersecting or overlapping in the diagram which makes explicit their relationship as a basis for any organization. As such, the diagram is somewhat trivial. However, the Venn diagram convention can be adapted in a useful way by introducing the concept of dominance or priority. (This is a simplistic assumption as is discussed later)

e.g. for 2 functions (see Fig 5)

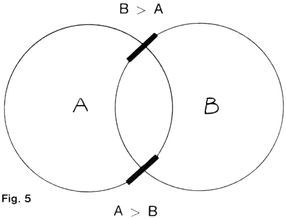By drawing thick lines at the cross-over points of the functional boundaries, it is possible to represent relationships of two distinct forms:

• function B has priority over function A
• function A has priority over function B

This may be interpreted from the diagram in terms of how the thicker line reflects either:

• the "penetration" of one boundary by the other, (e.g. A over B) or
• the "blockage" of any such penetration by an "impenetrable" boundary

This approach has the advantage of rendering explicit two functional situations of operational (or policy) significance. It thus goes beyond the relatively simplistic recognition of "A and B are interrelated If. In contrast to the Venn diagram convention, it is not the bounded area which denotes the function, but rather the boundary line or track (which most economically takes a circular form).The set of functions as initially conceived may give rise to misunderstanding if:

• each function is not of equal value (e.g. such that one is in all cases of lower priority than another)
• one does not in fact relate to the others in a group, or only relates to some of them

These possibilities are considered in a later section. If the set has been well-conceived, it would appear that its constituent functions should obey the following rule, provisionally formulated as:

Each function basic to the operation of a well-balanced group gives rise to two operational situations in relation to each other basic function:

• a situation in which it is of primary concern
• a situation in which it is of secondary concern.

Within the above convention, this is represented by patterns of thickened lines at the boundary cross-over points.

e.g. for 3 functions (see Fig. 6)

Maintaining equilibrium

While such functional "weaving" is interesting, it does not yet clarify what relationships are necessary to prevent operational shocks from de-stabilizing the configuration as a whole. What ensures the maintenance of dynamic equilibrium ? (Growth and evolution need to be considered later).

At this point it is helpful to conceive of the "functional boundary lines" as being at a higher level of abstraction than the thickened portions at the cross-over points. In effect the boundary lines are made manifest or defined by the thickened portions. The boundary lines may perhaps be considered "virtual memory". It is also helpful to conceive of the thickened portions as being straight, such that the curvature of the boundary must be defined by a series of thickened lines angled in relation to each other.

The necessary relationships may now be understood as the connectors required to protect the geometry defined by the thickened portions (separators) of the lines

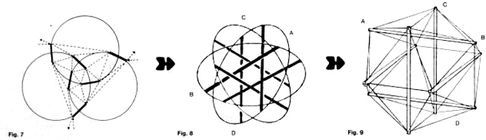
e.g. for 3 functions (see Fig. 7)

Inserting the first 12 connector lines demonstrates the approach to maintaining the configuration. But is also raises the very interesting questions of the unconnected ends of the separators and the limitations of the two-dimensional representation. An "outer" set of 9 connector lines way be added with some form of distortion, but this still leaves the "outermost" 3 separators incompletely bound compared to the others.The operational significance of such connector lines in an organisation will be discussed below. At this point it is sufficient to suggest that the incomplete bonding is characteristic of many (non-self-reliant) organisations which depend upon their external environments to maintain their functional stability. Typically this may involve either of the following:

• an external resource pool
• an external waste product "sink" (i.e. no recycling)

This dependence limits the organization's ability to recognize the process cycles through which it is embedded in, and "feeds" off, its environments. David Bohm's concept of "holocyclation" relates to this (4).

3-Dimensional equivalent

Because of the topological nature of the representation above, it can be transformed into a three-dimensional structure by simply "folding up the petals" in order to insert the 3 final connectors. Were the connectors made of elastic material, they would then become of equal length in such a final dynamic equilibrium configuration. Fig. 9 is thus the equivalent of Fig. 7. Fig. 9 is one of the simplest tensegrity structures: a tensegrity icosahedron. (Fig. 8 is an indication of how Fig. 9 approximates to the circles of Fig. 7). It would be a mistake to treat such representational transformations as trivial, for in operational terms they reflect a fundamental change in attitude in which "inner" and "outer" environments are recognized as inverted mirror reflections of each other. (In psychoanalytical terms, it is equivalent to an individual's confrontation with his own "shadow" and an understanding of the death/rebirth cycle - a counterpart to recognition of both the ultimate feedback consequences of waste-product dumping and the need for recycling).

It would also be a mistake to fail to reflect on the operational significance of the minimum opposing (complementary extreme) properties that need to be attributed to the "separators" (e.g. rigidity) and the "connectors" (e.g. flexibility) for the structure to exist. This has been touched on in earlier papers, but remains a fruitful area of exploration in which premature closure, even if possible, should be avoided. It raises the same difficulties as comprehending the nature of yin and yang in Chinese philosophy.

More than 3 functions

Clearly the same approach may be used where 4 or more functions are considered basic. In Fig 10, the case of 6 functions is explored. This results in a tensegrity icosidodecahedron. Six functions have been selected for the recently launched Hexiad Project, although the instigators choose to work with a different tensegrity structure in the light of an interesting alternative interpretation(5).

Now although this approach could be used in the case of any number of basic functions, there is an indication of an early limit. Most tensegrity structures have triangular, square, pentagonal or hexagonal "faces". Above this number there are problems of stability (which remain to be explored). Aside from which, in an organizational setting there are problems of handling more than about 7 varieties of information (1).

This limit may be bypassed by a logical extension of the method described above. This is explored in Fig. 12 for the case of the 26 sub-projects of the United Nations University's Goals, Processes and Indicators of Development project. By coincidence, a recently produced diagrammatic representation of the structure of that project reflects the first step in the extension of the method (see Fig. 12). Note that where the number of equivalent basic functions is so high, the circuits do not all interact with each others. In geometrical terms they are then lesser rather than great circles around the sphere; under certain conditions both may occur together. Although there are always the two forms of interaction. The rule mentioned above must therefore be modified to ensure adequate functional "basket weaving" for the "basket" to be a viable structure. Further investigation is required.

Functional articulation may be continued. The tensegrity structural consequence is reflected in the generation of geodesic polyhedra developed by R Buckminster Fuller(6). Needless to say, there are many interesting aspects, variants and exceptions (e.g. for less than 3 functions) which remain to be explored.

An important alternative to note emerges as the number of crossover points (or separators) on any circuit increases. Whilst the straight separators may always be reduced in length to maintain a separation between them (resulting in a "diamond pattern"), they may also be increased in length and allowed to touch (resulting in a "circuit pattern"). This eliminates the need for some connectors which then follow the circuit pattern of the linked separators (2). This can result in viable structures even when there are only three elements in the circuit. It is however the most common pattern with higher numbers of cross-over points per circuit.

Operational significance

For any of this to be of operational significance, the "recodification" of the basic features of such structures must be clarified:

1. Separators: It was suggested above that the abstract "functions" should be defined at the level of the separators which themselves only occur at interaction points between functions. As such they may be interpreted as some form of action in terms of one function but in relation to another. In earlier papers the notion of a "struggle" between opposing conceptions was suggested, and it is interesting that Callaway treats them as "projects" (5). It is a question of what one function has to do to define itself in relation to another. Thus, for example, in the interaction in an enterprise of the functions "human relations" and "production":

• human relations must dominate production under certain conditions (to which trade unions and personnel managers are particularly sensitive)
• production must dominate human relations if "the job is going to get done in time".

2. Circuits: The significance of circuits as functions has been discussed above. It needs to be stressed that functions cannot be described in the abstract or in isolation. They acquire reality in terms of action and that action only occurs in relation to other functions. (One image which may he helpful is that of a line of "boats" (separators) travelling around the circuit and meeting successive "waves" corresponding to other functional circuits. The separator denotes the special action required in each case for the direction (and functional integritv) to be maintained.

Fig. 10: Icosidodecahedron tensegrity represented in 2-dimensions

Case of 6 functions: Using the same approach as for Fig. 7, circles A, B, C, D, E and F can be interlinked to form Fig. 10. This is a two-dimensional representation of the tensegrity icosidodecahedron shown in Fig. 11 in three dimensions. The shaded pentagons in Fig. 10 are distorted progressively away from the centre but are all the same size in Fig. 11, as are the shaded triangles. The edges of these areas are the connectors. The separators are shown in thickened black lines, again distorted in length away from the centre. The five outermost shaded portions fold up together (like petals) to form the twelth pentagon. (Note that right- and left-handed versions of such tensegrities may be constructed. Comments on the lettering are given in connection with Fig. 12.

Fig. 11: Icosidodecahedron tensegrity in, 3-dimensions

3. Connectors: As suggested above, these may be taken to signify the lines of communication whereby the relationship between different activities (separators) is maintained in order to preserve the interrelationship between functions necessary for the functional integrity of the whole.

4. Facial areas: These are the triangular, square, pentagonal or hexagonal areas defined by the connectors (and also by the separators in the circuit pattern). They may be usefully thought of as "decision arenas" , possibly "roundtables" in which a balance has to be struck between the different forces (functional issues) entering the "mandate" of the particular arena. Clearly each such arena has quite distinct responsibilities. Note that the limitation on the number of sides to an area usefully reflects the practical limitations on the size of a viable committee or task force - or the varieties of information which can be handled together in such a setting.

5. Structural "pimples" and "dimples": There is no need to focus solely on spherically symmetrical structures, despite their advantages. Portions of a structure may be missing, resulting in a "dimple" (an expression used by Buckminster Fuller). This would correspond to unactivated functions and activities. On the other hand "extra" functions may be associated with the basic pattern as represented by elements added onto the spherical structure. This is a rich area for exploration.

Organizational significance

It is important to note that the organizational pattern has been defined above in terms of the realism of activities, functions, communications and decision "arenas". This contrasts with the formalism of conventional preoccupation with organisational units, reporting lines, and programmes.In the light of the preceding sections, it is now possible to map out the complete functional organization. This done, there is then the interesting question of the "organization structure" to be associated with each component. Some types of organization structure are:

• independent organization
• department/section
• programme/project
• person/role
• job activity
• attention time
• procedure/rule
• automated decision procedure.

Now the notion of function may, for example, be associated with a department, a programme, or a person, which would thus be represented by a circuit of separators. The attribution would depend on the complexity of the organization. Alternatively, an individual activity might be associated with a department, a project, or someone's attention time, which would thus be represented by a separator. A decision arena might be the responsibility of a committee or task force, a person, someone's attention time, or an automated decision procedure - again depending on the complexity of the organization.

The functional requirements make clear the activities or decision arenas to which attention must be given by whatever appropriate structural element - in order for the functional integrity of the organization to be maintained. In particular it draws attention systematically to features which have not been considered explicitly - and which certainly do not emerge from conventional organization charts. The non-linearly structured interrelationship of decision arenas here contrasts with the linear listings and checklists to be found in standard management textbooks. In the latter it is assumed that functional integrity is maintained by top-down decision making. The weakness of this is evident from the increasing number of constraints (e.g. in personnel relations) to which top-down management is subject.

In the tensegrity organization, the management/leadership function is merely one amongst several functional circuits. It dominates each other function under certain conditions, but is dominated by each of them under others. It is interesting that sophisticated hierarchical organizations achieve balance by having a functional safety-valve in which the "chief" is ridiculed and the "underdog" may even takeover temporarily. This is evident in some folk rituals but also occurs in rudimentary form in some modern corporations (e.g. annual celebrations in which custard pies may be thrown at the president, possibly at a price for a charitable cause; or the special freedoms of New Year parties). Where there are many functions, it may not even interact with some of them.

Implications

This paper indicates how, from the initial logical conception of an interrelated complex of functions, a non-hierarchical organizational structure can be elaborated. It has the merit of indicating specifically and systematically the interrelated operational concerns which must be borne in mind (or reflected in functional responsibilities) in order for functional integrity to be maintained. This is a significant structural and operational step beyond any checklist of "management do's and don'ts" or of a mathematically generated set of possible functional combinations.

The approach provides a functional coding scheme which emphasizes functional integrity in a non-parasitical relationship to the environment. As such it has built in "organic" and holistic qualities which contrast with the somewhat mechanical and fragmented thinking reinforced by conventional hierarchical coding schemes. It is almost as though the latter stressed a functional "flat earth" quality in contrast to functional "roundness" as a desirable alternative.

Whilst the approach lends itself to simple "cook-book" organisational design, any structures or structural features are a standing challenge inviting deeper comprehension. This is because the nature and significance of each element is necessarily determined by its relation to the functional whole. Thus if a verbally defined project is associated with a particular separator, the position of the separator is an invitation to understand a deeper significance of the words describing the project, or alternatively to recognize the functional characteristics the words have failed to capture.

The approach has much to recommend it in showing part/whole relationships such as of small groups (commissions) to a plenary body (e.g. in a meeting). It also offers a valuable means of seeing the relationship of independent organisations in a functionally integrated network.

In seeking to use tensegrities, it may prove that it is more appropriate to treat them as extremely powerful conceptual "interrelators"for whatever projection onto them can be adequately sustained and comprehended within the collectivity concerned. This may be incomprehensible to "outsiders If, thus unable to work within that pattern. It is useful, for example, to reflect on the elegance with which tensegrities can interrelate complex patterns of differences (separators) and similarities (connectors) at a time when society is torn between the simplistic extremes of personal or collective violence on the one hand, and sexual/ecstatic merger or planetary integration/union on the other. Could it be that tensegrities indicate a way of articulating and interrelating differences such that the latters' separative properties (normally destructive) provide the necessary basis for the construction of a new collective space ? (Is any attempt to base new structures solely on similarities doomed to failure as a contradiction in terms ?)

The tensegrity, as a configuration of differences, then provides a way of releasing and channelling energy from differences (in orientation, including opposition, for example). What then are the energy containing patterns of differences and similarity (cf. social differences and the similarities of "equality" paradigmatic and ideological differences, etc), and how can they evolve ? Is the energy derived through "oscillation" in relation to a more fundamental field than that embodied in the tensegrity as collectively comprehended ?

Finally, there is a certain practicality and elegance in moving beyond the divide-and-rule exploitation of the rivalries, which tend to undermine every initiative, to their transformation into a non-hierarchical structuring device - rather than trying vainly to eliminate them and thus forfeiting the energy they generate. The same might be said of the many illusions by which individuals and groups are plagued according to their learning experience - the containment and boundedness they provide, and the energy they generate, could well determine their place as information processing stages in a larger pattern which they collectively make manif est. Given the knowledge and population explosions, the amount of ignorance "generated" and "accumulated" is progressively increasing. If it cannot be eliminated, efforts should be made to benefit collectively from its characteristics before these make it impossible to do so. This perspective has the merit of indicating how this might be done without exploitation.

Although much remains to be investigated, numerous possibilities for practical experiment are now open. The Hexiad Project is to be congratulated on being the first to use tensegrity organization principles (5, 7). That they should be first used to link the alternative communities of Findhorn (Scotland), Auroville (India), and Arcosanti (USA), with the aid of computer conferencing techniques, is perhaps a lesson to more conventional international bodies.

Fig. 12: Diagrammatic representation of UNU/GPID structure Case of "26 functions"

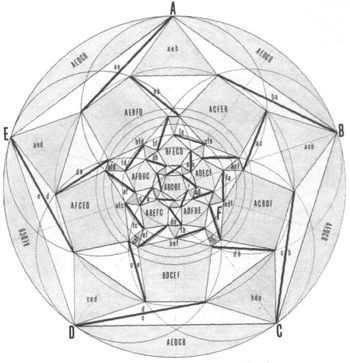
The Goals, Processes and Indicators of Development (GPID) project of the UN University started in 1977 with 24 subprojects (gradually increased by some 5 additional "study groups" of sub-project status). This complex project has recently been represented by Fig. 12 in which the small circles indicate 26 subprojects. There is internal concern regarding (a) proposals to increase the number of sub-projects, and (b) problems of ensuring appropriate interlinkage between them to constitute a coherent whole consistent with the GPID mandate. Assuming that each sub-project represents a distinctive "function", it is possible to construct, by geodesic subdivision of an icosahedron, a tensegrity with 30 functional circuits (some of them lesser circles). The (10-frequency) tensegrity icosahedron has 750 separators (activities ?) and 1500 connectors (communication paths ?). This seems to be unnecessarily complex given the level of activity of GPID. It is already difficult enough to understand. Assuming that the sub-projects are in fact "groupable" into sets of more basic "functions", a simpler approach may be sought. And in fact the original 24 were grouped into 4: goals, processes, indicators and tools. These however excluded the steering/coordinative function evident from Fig. 12. They also exclude the self-reflexive function characterized by some recent papers. For the sake of illustration only, let it be assumed that there are currently 6 basic functions grouping some 30 sub projects. In which case Fig.10 mav be used to explore the necessary interrelationships for the coherence of GPID as a whole. The separator circuits A, B. C, D, E and F represent the 6 functions with 5 sub-projects per circuit.For the GPID to maintain functional integrity, communications must then be maintained according to the pattern of connectors outlining the shaded areas of pentagons and triangles. The roundtable decision arenas represented by the latter are an indication of the interparadigmatic considerations to be maintained in balance. Some may appear obvious (possibly due to their ease or appeal), others much less so. The areas have been labelled according to the functions defining them. Thus area ABCDE (in the centre) indicates a priority or causal relationship of A>B>C>D>E>A... It may be considered as labelling the circularity of the priorities governed by a particular feedback loop necessary to the functional integrity of the GPID preoccupations.Note each labelled area is matched by one in which the reverse ordering holds. So ABCDE is matched by one formed when the outer areas are folded up to form the twelfth pentagon AEDCB. In the 3-dimensional form the apparent centrality of ABCDE in the 2-dimensional form is shown to be arbitrary (or at most the temporary consequence of a particular viewpoint). On the practical question of how to deeode the functions and their 30 sub-projects, Fig. 10 raises an important issue. Because each element is defined in relation to the whole, the conventional practice of only articulating any such definition within a sub-project is shown to be inadequate (or even dysfunctional). The significance of verbal descriptors is challenged by the context. It is in this light that matching of Fig. 10 to GPID elements should be explored. Are subprojects associated with a particular function equally distinct ? Is the set complete ? Into what "gaps" do additional sub-projects get slotted ? Are some sub-projects effectively sub-subprojects? Is there a functionally significant sequence to sub-projects associated with a particular function ? The art of reaching an appropriate balance is perhaps somewhat analogous (in their cultural contexts) to Navaho sand-painting or the associated discipline of mandala construction. Here however it is a collective exercise. As stressed earlier, there are many tensegrities and perhaps many ways of making use of their power as "interrelators". ln the future, sets of viable patterns for consideration could be generated by computer. But to exist at any given moment, a particular pattern must be used the act of distinguishing such a number-governed pattern is analogous to the cathedral builders choice of a set of n "sacred numbers" to govern the proportions and symmetry of the building Here the basic symmetry is a guarantee of non-hierarchical functional integrity. This is specially relevant to the continuing evolution of GPID through any more appropriate patterns of functional interlinkage. The dynamics of the relationships basic to the equilibrium of a model such as Fig. 11 will he considered in a later paper. But to think of it as static would be as misleading as crude portrayals of models of the Bohr atom or the DNA molecule.

References

1. Anthony Judge. Representation, comprehension and communication of sets; the role of number. International Classification, 5,1978, 3, pp. 126-133 (Pt. D; 6, 1 g79, 1, p 15-25 (Pt.ll); 6, 1979, 2, pp. 92-103 (pt 111)

2. Anthony Judge. Transcending duality through tensional integrity; from systems-versus-networks to tensegrity organization. Transnational Associations, 30, 1978, 5, pp. 258-265

3. Anthony Judge. Groupware configurations of challenge and harmony; an alternative approach to alternative organization, Transnational Associations, 31, 1979, 10, pp. 467-475.

4. David Bohm. Fragmentation in science and society. Impact of Science on Society (Unesco), 22, 2, 1970, pp. 159-169.

5. Peter Callaway. Introduction to tensegrity organization principles. Transnational Associations, 31, 1979, 12, pp. 592-599.

6. R. Buckminster Fuller with E. J. Applewhite. Synergetics: explorations in the geometry of thinking. Macmillan, 1975.

7. Jerome Clayton Glenn. Linking the Future; Findhorn, Auroville, Arcosanti. Hexiad Project, 1979.